ABC is an isosceles triangle with AB = AC. We extend the segment BA to D such that BA = AD. What is the value of ∠BCD?
Answer:
90°
- Take a look at the image below:
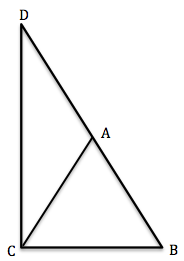
ABC is an isosceles triangle with AB = AC,
therefore, ∠ABC = ∠ACB = x
Let ∠CAB = y. Then y + x + x = 180°
⇒ y + 2x = 180°
⇒ ∠ACB = x =180°-y 2 - Since we extended BA to form line BD, ∠BAC + ∠DAC = 180°
⇒ y + ∠DAC = 180°
⇒ ∠DAC = 180° - y - CAD is also an isosceles triangle since AD = AC (remember AD = AB and AB = AC)
So ∠CDA = ∠DCA = z
⇒ ∠CDA + ∠DCA + ∠DAC = 180°
⇒ z + z + (180°-y) = 180°
⇒ 2z = y
⇒ ∠DCA = z =y 2 - Now, ∠DCB = ∠DCA + ∠ACB =
+180°-y 2
= 90°y 2
 Ireland
Ireland
